What are power reducing formula in Vietnam? When do students in Vietnam learn power reducing formula?

What are power reducing formula in Vietnam?

The power reducing formula is an important tool in trigonometry. It helps us convert higher-degree trigonometric expressions (like sin²x, cos²x, ...) into simpler forms, usually first-degree expressions involving cos or sin. This is very useful in solving trigonometric equations, calculating integrals, or simplifying complex expressions.

*Note: The information is for reference only./.

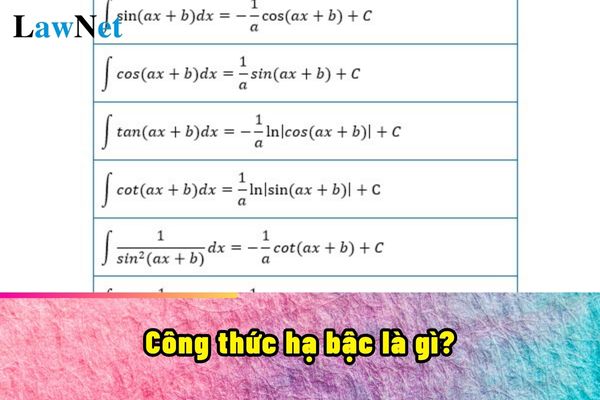
What are power reducing formula in Vietnam? When do students in Vietnam learn power reducing formula?​ (Image from the Internet)

When do students in Vietnam learn power reducing formula?

Accordingly, the power reducing formula is an important tool in trigonometry. It helps us convert higher-degree trigonometric expressions (like sin²x, cos²x, ...) into simpler forms, usually first-degree expressions involving cos or sin.

Thus, based on Section V of the High School Mathematics Curriculum issued with Circular 32/2018/TT-BGDDT, the requirements for the Grade 11 Mathematics program are as follows:

In the Algebra section, the lesson on Trigonometric Functions and Trigonometric Equations will include the following content:

- Trigonometric angles, Measurement of trigonometric angles, Trigonometric circle, Trigonometric values of trigonometric angles, relationships between trigonometric values, Trigonometric transformations (addition formulas; double-angle formulas; product-to-sum formulas; sum-to-product formulas), with the following requirements:

+ Recognize the basic concepts of trigonometric angles: the concept of trigonometric angles; measurement of trigonometric angles; the Chasles relation for trigonometric angles; the trigonometric circle.

+ Recognize the concept of the trigonometric value of a trigonometric angle.

+ Describe the table of trigonometric values for common trigonometric angles; the basic relationships between trigonometric values of an angle; relationships between the trigonometric values of particularly related angles: complementary, supplementary, opposite, differing by a certain amount.

+ Use a scientific calculator to compute the trigonometric value of an angle when its measure is known.

+ Describe basic trigonometric transformations: addition formulas; double-angle formulas; product-to-sum formulas; sum-to-product formulas.

+ Solve some practical problems related to trigonometric values of angles and trigonometric transformations.

- Trigonometric functions and graphs requirements:

+ Recognize concepts of even functions, odd functions, periodic functions.

+ Recognize geometric characteristics of graphs of even functions, odd functions, periodic functions.

+ Recognize definitions of the trigonometric functions y = sin x, y = cos x, y = tan x, y = cot x through the trigonometric circle.

+ Describe the value tables of these four trigonometric functions over a period.

+ Draw graphs of the functions y = sin x, y = cos x, y = tan x, y = cot x.

+ Explain the domain; range; parity; periodicity; periods; intervals of increase and decrease of the functions y = sin x, y = cos x, y = tan x, y = cot x based on their graphs.

- Basic trigonometric equation requirements:

+ Recognize solution formulas of basic trigonometric equations: sin x = m; cos x = m; tan x = m; cot x = m by using the corresponding trigonometric function graphs.

+ Calculate approximate solutions of basic trigonometric equations using a scientific calculator.

+ Solve trigonometric equations by applying basic trigonometric equations directly (e.g., solve trigonometric equations like sin 2x = sin 3x, sin x = cos 3x).

+ Solve some practical problems related to trigonometric equations (e.g., problems related to harmonic oscillation in physics, ...).

Thus, in the Grade 11 Mathematics program, students will learn and become familiar with the power reducing formula to solve problems related to solving trigonometric equations.

What are regulations on the evaluation of educational results in Mathematics under the new curriculum in Vietnam?

Based on Section 7 of the High School Mathematics Curriculum issued with Circular 32/2018/TT-BGDDT, the evaluation of educational results in Mathematics under the new curriculum is as follows:

Applying a combination of multiple assessment forms (process evaluation, periodic evaluation), various assessment methods (observation, recording implementation process, oral questioning, objective tests, written tests, practical assignments, projects/products, real-world tasks, ...) at appropriate times.

Process evaluation (or continuous assessment) is organized by the subject teacher, combined with evaluations from teachers of other subjects, self-evaluations by the students being assessed, evaluations by peers in the group, class, or evaluations by parents. Process evaluation is integrated with the progress of students' learning activities, avoiding the separation between teaching and assessment, ensuring the goal of evaluation for students' progress in learning.

Periodic evaluation (or summative assessment) mainly aims to assess the achievement of learning goals. Results of periodic and summative assessments are used to certify levels of learning, recognize students' achievements. Periodic evaluation is organized by educational institutions or through national exams and assessments.

Periodic assessment also serves to manage teaching activities, ensure quality at the educational institution, and support the development of the Mathematics curriculum.

Assessing students' competencies through evidence of results achieved in the process of performing student actions.

The assessment process includes basic steps such as defining the purpose of assessment; determining necessary evidence; choosing appropriate methods and tools for assessment; collecting evidence; interpreting evidence and providing feedback.

Focusing on selecting methods and tools to evaluate components of mathematical competencies. To be specific:

- Evaluating mathematical thinking and reasoning ability: methods and tools such as questions (spoken or written), exercises,... requiring students to present, compare, analyze, aggregate, systematize knowledge; apply mathematical knowledge to explain and reason.

- Evaluating mathematical modeling ability: choose real-life situations that give rise to mathematical problems.

From there, students must identify a mathematical model (including formulas, equations, tables, graphs, ...) for the situation in the mathematical problem; solve the mathematical problems within the established model; interpret and evaluate the solution in the real-world context and improve the model if the solution is not appropriate.

- Evaluating problem-solving ability in mathematics: methods such as asking students to identify situations, detect and present the problems to be solved; describe, explain the initial information, objectives, and desires of the problem situation being considered; collect, select, organize information and connect with existing knowledge; use questions (requiring oral or written responses) that require students to apply their knowledge to solve problems, especially real-world problems;

Also using observational methods (e.g., checklists according to predetermined criteria), observe students in the process of problem-solving; evaluate through students' practical products (e.g., products of learning projects); reasonably consider evaluation tasks that are integrative.

- Evaluating mathematical communication ability: methods such as asking students to understand and interpret spoken or written texts, summarize, analyze, select, and extract basic and central mathematical information; use mathematical language in combination with everyday language to present, express, raise questions, discuss, and argue mathematical content, ideas, and solutions in interaction with others.

- Evaluating the ability to use mathematical tools and instruments: methods such as asking students to recognize the names, functions, usage guidelines, maintenance methods, advantages, and limitations of mathematical tools and instruments; present how to (appropriately) use tools and instruments to perform learning tasks or express mathematical reasoning and proofs.

When teachers plan lessons, they need to establish criteria and assessment methods to ensure that at the end of each lesson, students achieve the basic requirements based on the established criteria before proceeding to the next learning activities.