What are antiderivatives formulas? In which educational level in Vietnam will the antiderivatives formulas be taught?
What are antiderivatives formulas?
The antiderivatives is a fundamental concept in mathematics, especially in the field of calculus. It represents a family of functions whose derivative is a given function.
Definition: For a function f(x), a function F(x) is called an antiderivatives of f(x) over an interval K if F'(x) = f(x) for all x belonging to K.
Notation: We denote the set of all antiderivatives s of f(x) as ∫f(x)dx.
| antiderivatives Formulas *General Formula There is no single formula for all functions, but we have antiderivatives formulas for some basic functions: - Constant function: ∫kdx = kx + C (where k is a constant) - Power function: ∫x^n dx = (x^(n+1))/(n+1) + C (where n ≠ -1) - Exponential function: ∫a^x dx = (a^x)/ln(a) + C (where a > 0, a ≠ 1) - Logarithmic function: ∫(1/x)dx = ln |
x | + C - Trigonometric functions, including: ∫sin(x)dx = -cos(x) + C ∫cos(x)dx = sin(x) + C ∫(1/cos^2(x))dx = tan(x) + C ∫(1/sin^2(x))dx = -cot(x) + C ... (many other formulas) *Properties of antiderivatives s Linearity property: ∫[f(x) + g(x)]dx = ∫f(x)dx + ∫g(x)dx ∫k.f(x)dx = k∫f(x)dx (where k is a constant) *Methods of Calculating antiderivatives s: - For more complex functions, we need to use integration methods such as: - Integration by parts: Used when the integrand is the product of two functions. - Substitution method: Used when expressing an expression in the function by a new variable. - Rational fractions integration: Used when the integrand is a rational function. * Example: Find the antiderivatives of the function f(x) = 2x + 3. Solution: ∫(2x + 3)dx = 2∫xdx + 3∫dx = x^2 + 3x + C |
|
Antiderivatives Formulas *General Formula - Trigonometric functions, including: ∫sin(x)dx = -cos(x) + C ... (many other formulas) *Properties of antiderivatives s |
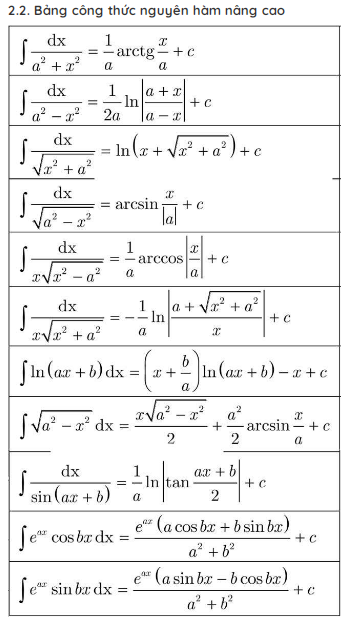
*Note: The information is for reference purposes only./.
When do students in Vietnam learn the antiderivatives formula?
According to Section IV of the Mathematics curriculum issued under Circular 32/2018/TT-BGDDT, the requirements in the Mathematics curriculum for grade 12 include:
Antiderivatives - Integral, wherein:
Antiderivatives. The antiderivatives table of some elementary functions
- Understanding the concept of the antiderivatives of a function.
- Explaining the basic properties of antiderivatives s.
- Identifying the antiderivatives of certain elementary functions such as:
y = xα (α ≠ -1); y = sinx; y = sinx; y = cosx; y = ax; y = ex.
- Calculating the antiderivatives in simple cases.
Therefore, the antiderivatives formula is taught in the grade 12 mathematics curriculum.
What are antiderivatives formulas? In which educational level in Vietnam will the antiderivatives formulas be taught? (Image from the Internet)
What are the teaching methods for grade 12 Mathematics in Vietnam?
Based on Section VI of the Mathematics curriculum issued under Circular 32/2018/TT-BGDDT, the curriculum for Mathematics in general and for grade 12 Mathematics specifically will employ the following methods:
- The teaching methods in the Mathematics curriculum must meet the following basic requirements:
+ Suit the cognitive process of students (from concrete to abstract, from easy to difficult); focusing not only on the logical nature of mathematical science but also on an approach based on students' experience and learning.
+ Adhere to the principle of “student-centered learning”, enhancing engagement and self-awareness, considering the needs, cognitive abilities, and different learning methods of each student; organizing the teaching process in a constructive direction where students are involved in exploring, discovering, reasoning, and solving problems.
+ Be flexible in applying active teaching methods and techniques; creatively combine traditional teaching methods and techniques with active teaching techniques; blend classroom activities with experiential, practical activities to apply mathematical knowledge in real life. The structure of the lesson should ensure a balanced proportion between core knowledge, application knowledge, and other components.
+ Use adequate and effective teaching tools and equipment as defined for the subject of Mathematics; possibly use self-made teaching aids suitable for the content and the students; increase the use of information technology and modern teaching tools appropriately and effectively;
- Orientation for methods to form and develop key competencies and general skills
+ Methods to form and develop key competencies
Through organizing learning activities, Mathematics, together with other subjects and educational activities, helps students train honesty, love for labor, a sense of responsibility, and a sense of fulfilling academic duties; nurturing confidence, interest in learning, habits of reading, and awareness of exploring and discovering science.
+ Methods to form and develop general skills
++ Mathematics contributes to the formation and development of self-regulation and self-learning ability by training learners to choose learning goals, make study plans, independently learn, reflect, and adjust to apply to different situations when learning mathematical concepts, knowledge, and skills as well as when practicing, solving problems or self-solving mathematical problems.
++ Mathematics contributes to forming and developing communication and cooperation skills through listening, reading, writing, interpreting necessary mathematical information in mathematical texts; effectively using mathematical language combined with standard language to exchange and present mathematical content, ideas, and solutions in interaction with others, demonstrating confidence and respect for interlocutors when describing and explaining mathematical content and ideas.
++ Mathematics contributes to forming and developing problem-solving and creative abilities by helping students recognize problematic situations; share their understanding of problems with others; propose, select methods, and processes to solve problems and present solutions; evaluate the implemented solutions and generalize to similar problems.
- Teaching methods for Mathematics contribute to forming and developing computational abilities, language skills, and other specific competencies. To be specific:
+ Mathematics, with its distinct advantages, has many opportunities to develop computational abilities, providing mathematical knowledge, practicing computational skills, estimating, and forming and developing elements of mathematical competency (analytic and reasoning skills, modeling abilities, problem-solving skills, communication skills, and the ability to use tools and means in learning mathematics).
+ Mathematics contributes to developing language skills by practicing reading comprehension, interpreting, analyzing, and evaluating situations with mathematical significance, through effectively using mathematical language combined with standard language to present, interpret content, ideas, and mathematical solutions.
+ Mathematics contributes to developing information technology skills by using IT tools and means as supporting tools in learning and self-learning; creating an experiential learning environment.
+ Mathematics contributes to developing aesthetic skills by helping students get acquainted with the history of mathematics, biographies of mathematicians, and recognizing the beauty of mathematics in the natural world.
Download to see the detailed Mathematics curriculum issued under Circular 32/2018/TT-BGDDT.
- Directive on National Resistance when Established? What Grade Level is 9th Grade?
- Vietnam: What is the overview of industrial revolutions over periods in the 10th-grade History curriculum? What knowledge about industrial revolutions do 10th-grade students learn?
- What is the Plan for organizing professional training for English teachers in Ho Chi Minh City about?
- Vietnam: What are the sample outlines of a social argumentative essay on kindness for 9th-grade students? What are the kindness qualities required for 9th-grade students?
- Vietnam: What are the sample argumentative essays on respecting people's differences for 11th-grade students? What are the conditions for 11th-grade students to be eligible for grade advancement?
- Are students pursuing dance in Vietnam eligible for tuition reduction?
- What are the standards for facilities of a Center for Continuing Education and Vocational Education in Vietnam?
- What are the conditions for a foreign educational institution to establish a representative office in Vietnam?
- What is the term of office of the principal of an intermediate school in Vietnam?
- What are the eligibility criteria for applying to an upper-secondary boarding ethnic school in Vietnam?
